Gears/gearing
Gears are used in thousands of mechanical devices ranging from precision wristwatches to automotive transmissions. The primary purpose of gears is to exchange speed for torque or vice-versa. This is important because, often, a small motor spinning fast can provide enough power for a device, but not enough torque. For instance, an electric screwdriver has a very large gear reduction because it needs lots of torque to turn screws, but the motor only produces a small amount of torque at a high speed. With a gear reduction, the output speed can be reduced while the torque is increased.
Another thing gears do is adjust the direction of rotation. For instance, in the differential between the rear wheels of your car, the power is transmitted by a shaft that runs down the center of the car, and the differential rotates that power 90 degrees to apply it to the wheels. [Source: How Stuff Works]
On most gear sets, the gear ratio is determined by the number of teeth each gear has. For instance, in a device with two gears, if one gear has twice the number of teeth compared to the other, the gear ratio would be 2:1.
One of the most primitive types of
gears we could look at would be a
wheel with wooden pegs sticking out of it. The problem with this type of gear is
that the distance from the center of each gear to the point of contact changes
as the gears rotate. This means that the
gear ratio changes as the gear turns, meaning that the output speed also
changes. If you used a gear like this in
your car, it would be impossible to maintain a constant speed -- you would be
accelerating and decelerating constantly.
Virtually all modern gears use a special tooth profile called an involute. This profile has the important property of
maintaining a constant speed ratio between the two gears. Like the peg wheel above, the contact point
moves, but the shape of the involute gear tooth compensates for this
movement.
Now let's take a look at some of the different types of gears.
Spur Gears:
Spur gears are the most common type of gears. They have straight teeth, and are mounted on parallel shafts, as shown in figure 1. Sometimes, multiple spur gears are used at once to create very large gear reductions. Spur gears are used in many devices, like the electric screwdriver, oscillating sprinkler, windup alarm clock, washing machine and clothes dryer. However, you won't find many in your car. This is because the spur gear can be quite noisy when transmitting power at high speeds. Each time a gear tooth engages a tooth on the other gear, the teeth collide, and this impact makes a noise. It also increases the stress on the gear teeth.

Figure 1: Spur gears
Helical Gears:
To reduce the noise and stress in the gears, most of the gears in your car are helical. The teeth on helical gears are cut at an angle to the face of the gear, as seen in figure 2. When two teeth on a helical gear system engage, the contact starts at one end of the tooth and gradually spreads as the gears rotate, until the two teeth are in full engagement. This gradual engagement makes helical gears operate more smoothly and quieter than spur gears. For this reason, helical gears are used in almost all car transmissions. Because of the angle of the teeth on helical gears, they create a thrust load on the gear when they mesh. Devices that use helical gears have bearings that can support this thrust load.

Figure 2: Helical gears
One interesting thing about
helical gears is that if the angles of the gear teeth are correct, they can be
mounted on perpendicular shafts, adjusting the rotation angle by 90
degrees. This is illustrated in figure
3.

Figure 3: Crossed helical gears
Bevel Gears:
Bevel gears are useful when the direction of a shaft's rotation
needs to be changed. They are usually
mounted on shafts that are 90 degrees apart, but can be designed to work at
other angles as well.
The teeth on bevel gears can be straight, spiral or hypoid. Straight bevel gear teeth have the same
problem as straight spur gear teeth -- as each tooth engages, it impacts the
corresponding tooth all at once. An
example of bevel gears is shown in figure 4 below.

Figure 4: Bevel gears
Just
like with spur gears, the solution to this problem is to curve the gear
teeth. These spiral teeth engage just
like helical teeth: the contact starts at one end of the gear and progressively
spreads across the whole tooth, as seen in figure 5.

Figure 5: Spiral
bevel gears
On straight and spiral bevel gears, the shafts must be perpendicular to each other, but they must also be in the same plane. If you were to extend the two shafts past the gears, they would intersect. The hypoid gear, on the other hand, can engage with the axes in different planes. This feature is used in many car differentials. The ring gear of the differential and the input pinion gear are both hypoid. This allows the input pinion to be mounted lower than the axis of the ring gear. Figure 6 shows the input pinion engaging the ring gear of the differential. Since the driveshaft of the car is connected to the input pinion, this correspondingly lowers the driveshaft. This means that the driveshaft doesn't intrude into the passenger compartment of the car as much, making more room for people and cargo.

Figure 6: Hypoid bevel gears in a car differential
Worm Gears:
Worm gears are used when large gear reductions are needed. It is common for worm gears to have reductions of 20:1, and even up to 300:1 or greater.
Many worm gears have an interesting property that no other gear set has: the worm can easily turn the gear, but the gear cannot turn the worm. This is because the angle on the worm is so shallow that when the gear tries to spin it, the friction between the gear and the worm holds the worm in place.
This feature is useful for machines such as conveyor systems, in which the locking feature can act as a brake for the conveyor when the motor is not turning. A worm gear set is shown in figure 7 below.

Figure 7: Worm gear
Rack & Pinion Gears:
Rack and pinion gears are used to convert rotation into linear motion. A perfect example of this is the steering system on many cars. The steering wheel rotates a gear which engages the rack. As the gear turns, it pushes the rack either to the right or left, depending on which way you turn the wheel. Rack and pinion gears are also used in some scales to turn the dial that displays your weight. Examples are shown below in Figure 8.

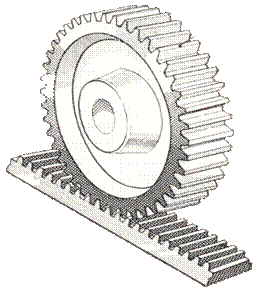
Figure 8: Rack & pinion gears from a bathroom scale
Gear Speed & Torque Relationships:
When two gears are in contact, the relationship between speed and torque can easily be computed. The peripheral velocity (V) at the point of contact must be equivalent to satisfy the “no-slip” condition.
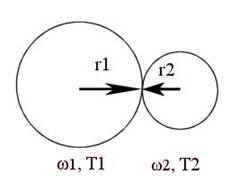
Equating the two velocities:
V1 = V2 => ω1 r1 = ω2 r2 => ω2 = ω1 r1 / r2
If we assume one gear is twice as large as the other:
If r1 = 2 r2 => ω2
= 2 ω1
Finally, performing a force balance on the two teeth in contact at any instant:
F1 = F2 => T1 / r1 = T2 / r2 => T2 = T1 r2 / r1
If r1 = 2 r2 => T2 = T1
/ 2
Video W1: How gears are made
Video W2: Gear basics
Wonderful cutaway models illustrating many of these concepts: https://web.archive.org/web/20150527214127/http://www.technolab.org/content/hako/en/index.php?lg=en&rg=en&cmp=hako&tpl=katalog&id=section10
Excellent video describing how car differential gearing functions:
http://www.youtube.com/watch?v=kJY9SxDOTog